블로그를 이전하였습니다. 2023년 11월부터 https://bluemiv.github.io/에서 블로그를 운영하려고 합니다. 앞으로 해당 블로그의 댓글은 읽지 못할 수 도 있으니 양해바랍니다.
반응형
1. 병합 정렬
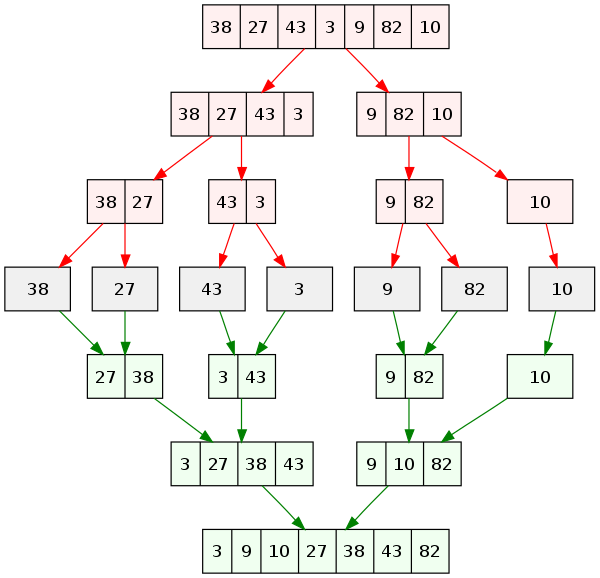
병합 정렬(Merge sort)은 그룹을 반으로 나눈다음 합칠때 크기에 맞게 합친다. 이 과정을 재귀적으로 수행한다. (그림 참고)

2. 코딩
아래 코드는 unittest 기반으로 작성되었다.
2.1. 일반적인 병합 정렬
<python />
# -*- coding: utf-8 -*-
import unittest
class Exam10(unittest.TestCase):
@classmethod
def setUp(cls):
pass
def test_merge_sort(self):
"""일반적인 병합 정렬"""
def _merge_sort(group):
# 재귀 종료 조건: 그룹이 없을때
n = len(group)
if n <= 1:
return
# 반으로 그룹을 나눈다.
mid_idx = n // 2
group1 = group[:mid_idx]
group2 = group[mid_idx:]
# print(group, group1, group2)
# 재귀적으로 병합 정렬을 수행한다.
_merge_sort(group1)
_merge_sort(group2)
# 정렬한다.
idx, idx1, idx2 = 0, 0, 0 # 전체 그룹 인덱스, 그룹1 인덱, 그룹2 인덱스
while idx1 < len(group1) and idx2 < len(group2):
if group1[idx1] < group2[idx2]:
group[idx] = group1[idx1]
idx1 += 1
else:
group[idx] = group2[idx2]
idx2 += 1
idx += 1
# 그룹에 남아있는 원소를 넣는다.
while idx1 < len(group1):
group[idx] = group1[idx1]
idx1 += 1
idx += 1
while idx2 < len(group2):
group[idx] = group2[idx2]
idx2 += 1
idx += 1
data = [38, 27, 43, 3, 9, 82, 10]
_merge_sort(data)
self.assertEqual([3, 9, 10, 27, 38, 43, 82], data)
if __name__ == "__main__":
unittest.main()
2.2. 파이썬을 이용한 병합 정렬
크게 차이점은 없다. _merge_sort() 가 return 값이 있는지 없는지에 대한 차이점이 있다.
<python />
# -*- coding: utf-8 -*-
import unittest
class Exam10(unittest.TestCase):
@classmethod
def setUp(cls):
pass
def test_merge_sort_with_python(self):
"""파이썬을 이용한 병합 정렬"""
def _merge_sort(group):
# 종료 조건 설정
n = len(group)
if n <= 1:
return group
# 그룹 나누기
mid_idx = n // 2
group1 = _merge_sort(group[:mid_idx])
group2 = _merge_sort(group[mid_idx:])
# 정렬
result = list()
while group1 and group2:
result.append(group1.pop(0) if group1[0] < group2[0] else group2.pop(0))
result.extend(group1)
result.extend(group2)
# print(result)
return result
data = [38, 27, 43, 3, 9, 82, 10]
sorted_data = _merge_sort(data)
self.assertEqual([3, 9, 10, 27, 38, 43, 82], sorted_data)
if __name__ == "__main__":
unittest.main()
이렇게 반으로 나누어서 문제를 푸는 방식을 분할 정복(Divide and Conquer)이라고 한다.
분할 정복은 시간복잡도가 O(N * logN) 이다. 선택 정렬이나 삽입 정렬의 시간 복잡도 O(N^2) 보다 낮아, 비교적 빠르다.
즉, 개수가 많아질수록 병합정렬(Merge Sort)을 이용하는것이 효율적이다.
다른 방법의 정렬 알고리즘은 아래를 참고한다.
- 2020/03/21 - [Algorithm] - 선택 정렬 (Selection Sort) 알고리즘 (with Python3)
- 2020/03/21 - [Algorithm] - 삽입 정렬 (Insertion Sort) 알고리즘 (with Python3)
- 2020/03/25 - [Algorithm] - 퀵 정렬 (Quick Sort) 알고리즘 (with Python3)
- 2020/03/25 - [Algorithm] - 버블 정렬 (Bubble Sort) 알고리즘 (with Python3)
3. Reference
반응형
'Algorithm' 카테고리의 다른 글
| 버블 정렬 (Bubble Sort) 알고리즘 (with Python3) (0) | 2020.03.25 |
|---|---|
| 퀵 정렬 (Quick Sort) 알고리즘 (with Python3) (4) | 2020.03.25 |
| 삽입 정렬 (Insertion Sort) 알고리즘 (with Python3) (0) | 2020.03.21 |
| 선택 정렬 (Selection Sort) 알고리즘 (with Python3) (0) | 2020.03.21 |
| 하노이탑 알고리즘 구현 (with Python3) (0) | 2020.03.17 |